From string theory to gauge theory - the AdS/CFT correspondence

The NCN Maestro grant From string theory to gauge theory - the AdS/CFT correspondence, directed by Romuald A. Janik has been running during the period 2013-2018. The research team included Andrzej Wereszczyński, also at the Jagiellonian University, as well as a team of postdocs employed specifically for the project: Ines Aniceto, Hesam Soltanpanahi, Michael Abbott, Mario Flory and Alvaro Veliz-Osorio. In addition, we benefitted from long-term collaborations with Jakub Jankowski (Jagiellonian University and University of Warsaw), Zoltan Bajnok (Wigner Research Center, Budapest), Michał Spaliński (NCBJ and University of Białystok) and Loredana Bellantuono (visited Jagiellonian University on an Angelo Della Riccia fellowship).

The AdS/CFT correspondence

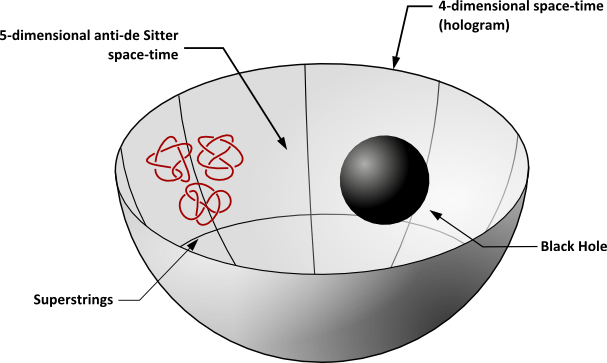image source: www.quantum-bits.org

The so-called AdS/CFT correspondence is a postulated equivalence (or duality) between two completely different kinds of theories: gauge theories in flat spacetime and string theory in specific higher dimensional curved spacetimes with boundary. The equivalence between the two kinds of theories means that physical problems in one theory can be translated into problems within the framework of the other theory. Due to the fact that the gauge theory can be understood as living on the boundary of the higher dimensional curved spacetime, the equivalence of the two theories is often referred to as holography. Since the theories on both sides of the duality are completely different, in particular string theory necessarily includes gravity, this offers the fascinating possibility of studying dynamical properties of gauge theories in terms of problems in higher-dimensional gravity. E.g. thermal properties of gauge theory plasma (like quark-gluon plasma observed at RHIC and LHC) can be mapped to properties of 5-dimensional black holes. An additional bonus of such a reformulation is that the most elusive and difficult to study nonperturbative regime of gauge theory corresponds to a tractable regime of classical strings/gravity.

The AdS/CFT correspondence can be studied from various perspectives. Firstly, one can use the aforementioned mapping to investigate real time dynamics in nonperturbative gauge theory using the dual gravitational language. This is especially important as for these types of problems we lack calculational methods on the gauge theory side. Secondly, one can use special properties of solvability (integrability) of string theory in AdS5xS5 spacetime to obtain exact results for an interacting 4-dimensional gauge theory (N=4 Super-Yang-Mills theory). Thirdly, one can aim to understand the microscopic origin of holography from the field theory perspective.

The research undertaken in the course of the grant concentrated predominantly on the first two perspectives but also explored the third direction in the final period of the project. Below we will present some selected research highlights obtained during the course of the NCN Maestro grant, giving links to the arXiv versions of the papers.

High order hydrodynamics

Hydrodynamics is a virtually universal way of describing systems close to thermal equilibrium in terms of local energy density, pressure and a flow velocity. It can be understood as a systematic gradient expansion, with the 0th order given by perfect fluid, the first subleading order including shear and bulk viscosity terms and of course a potentially infinite set of higher derivative terms. The structure of all those terms can be classified by symmetry, while the coefficients (so-called transport coefficients) depend on the microscopic details of the particular theory. As with any perturbative expansion, it is very intriguing to understand its overall behaviour like convergence etc. However, in order to do this, one has to go to high orders in the derivative expansion which includes specific values of the theory dependent transport coefficients, which, generically, are notoriously difficult to calculate. For this reason, these properties were never investigated. However this technical problem may be overcome using the dual gravitational description.

Using the gravity side of the AdS/CFT correspondence, we computed 240 terms in the late-time expansion of boost-invariant evolution of plasma in N=4 SYM. Each of these terms gets contributions from subsequently higher orders in the derivative expansion of hydrodynamics. The main observation was that the hydrodynamic gradient expansion is asymptotic and has zero radius of convergence.

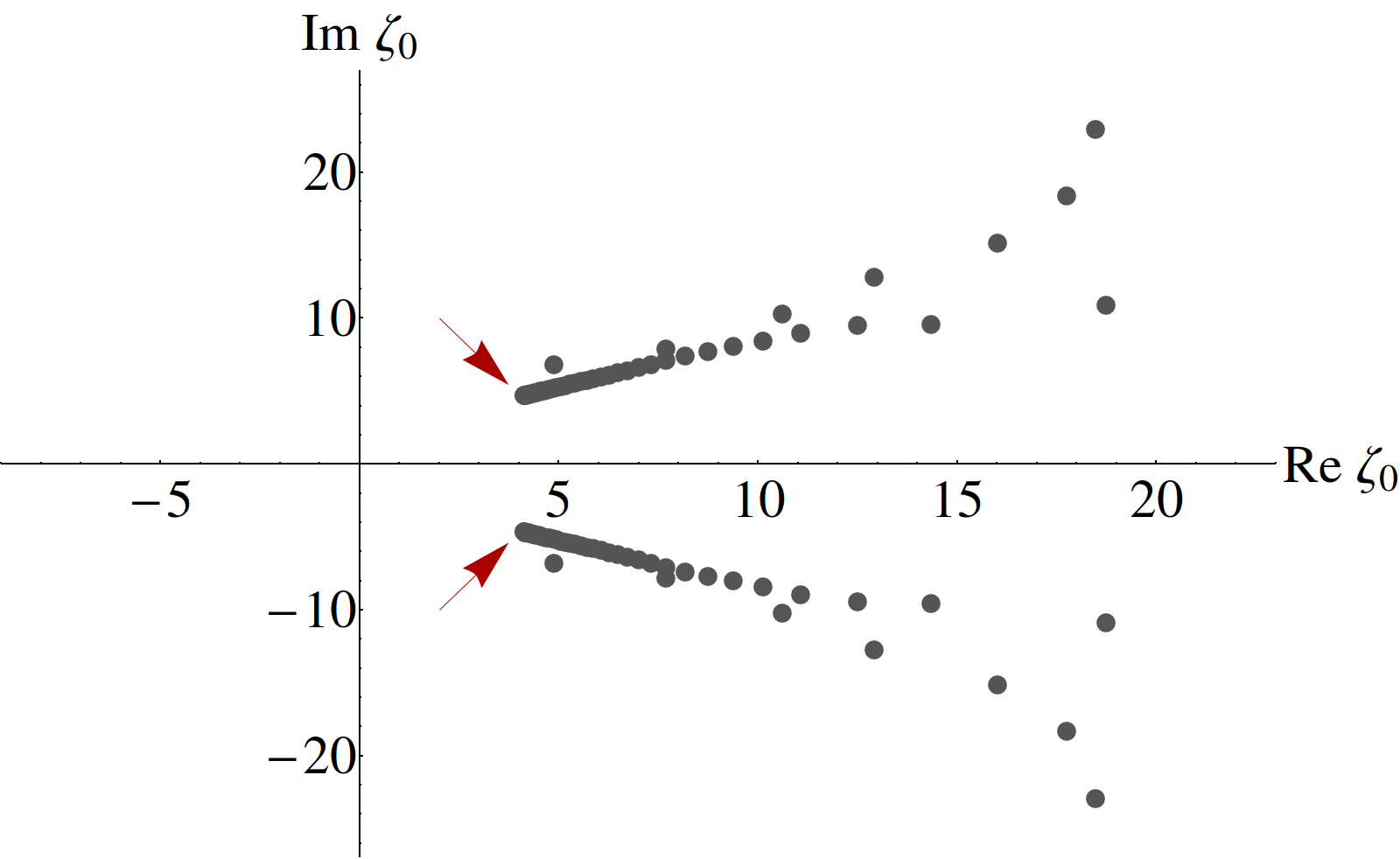
Once we have an asymptotic series, it is interesting to understand the physical origin of the nonconvergence. For standard perturbative series, this is given by instantons etc. In order to analyze this issue, we studied the singularities (of the Borel transformed series) in the Borel plane (see figure). The two cuts turn out to correspond exactly to the lowest non hydrodynamic collective excitations of the plasma, which manifest themselves on the gravity side as (non hydrodynamic) quasi-normal modes (QNM) of the 5-dimensional (expanding) black hole. The frequencies extracted from the cuts correspond with high accuracy to the results of a direct computation of QNM frequencies in General Relativity. The results of this work were published in M.P. Heller, R.A. Janik, P. Witaszczyk, Hydrodynamic gradient expansion in gauge theory plasmas, Phys. Rev. Lett. 110 (2013) 211602.

Quasi-normal modes - from generalized hydrodynamics to phase transitions

The breakdown of hydrodynamics occurs through the existence of non-hydrodynamic excitations, which manifest themselves on the gravity side through non-hydrodynamic quasi-normal modes. In contrast to the systematic construction of hydrodynamics, we do not know a-priori how these degrees of freedom evolve. One way would be to perform a 5D numerical relativity simulation on the dual side of AdS/CFT, but this is prohibitively complicated. In the paper M.P. Heller, R.A. Janik, M. Spaliński, P. Witaszczyk, Coupling hydrodynamics to nonequilibrium degrees of freedom in strongly interacting quark-gluon plasma, Phys. Rev. Lett. 113 (2014) 261601 we proposed a phenomenological extension of conventional hydrodynamics which incorporates also the non-hydrodynamic degrees of freedom corresponding to the lowest non-hydrodynamic QNM's. The extension is based on what we know about the QNM dynamics from holography, and it can be used for modelling quark-gluon plasma dynamics.

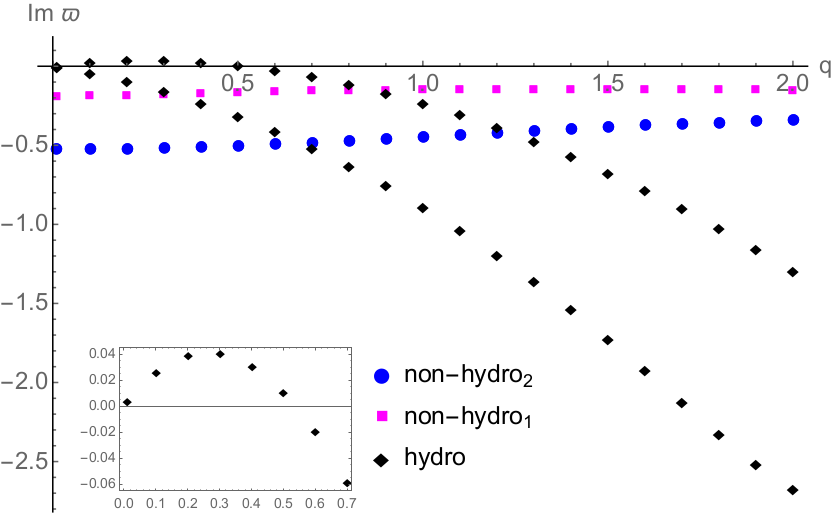
Since the spectrum of hydrodynamic and non-hydrodynamic quasi-normal modes provides important information on the dynamics of plasma away from equilibrium such as limitations on the applicability of hydrodynamics, potential instabilities etc., it is important to study the spectrum for theories in the vicinity of phase transitions of various kinds. Using holography we found a variety of new phenomena in comparison to the previously studied conformal case (see figure for the imaginary part of the frequency for a theory with a 1st order phase transition). We found that the nonhydrodynamic QNM's are less damped and hence decrease the applicability of hydrodynamics in the vicinity of phase transition. We observed the expected spinoidal instability (positive imaginary part of the frequency for a range of momenta - see figure inset) and, for some theories, a new kind of dynamical (nonhydrodynamic) instability. In addition, we observed a curious purely dissipative sound mode for a range of momenta in the deeply overcooled phase. These results were published in R.A. Janik, J. Jankowski, H. Soltanpanahi, Non-equilibrium dynamics and phase transitions, Phys. Rev. Lett. 117 (2016) 091603.

Phase separation in a holographic first order phase transition

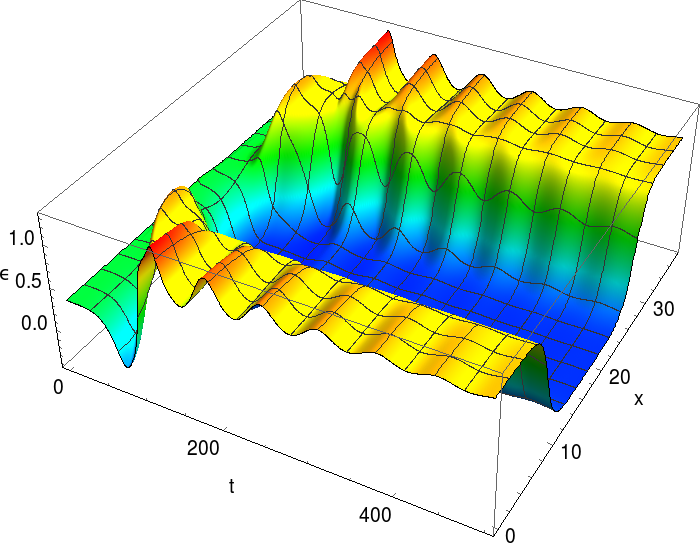
Quasi-normal modes describe only linearized dynamics around equilibrium. It is of great interest, although much more difficult, to study fully nonlinear out-of-equilibrium evolution. This is especially important when there exists an instability (like the spinoidal instability for theories with first order phase transition), as the determination of the end-point of the evolution requires nonlinear analysis. Due to holography this can be done on the gravity side by solving appropriate gravitational Einstein's equations.

Using these techniques, we have demonstrated, for the first time within holography, that at the endpoint of the spinoidal instability we obtain domains of the two stable coexisting phases (with equal free energies) at the first order phase transition separated by domain walls.

Of course, this behaviour is physically expected to occur at a first order phase transition. The benefit of holography is, however, that one can study the fine details of the whole process of domain formations, their collision and merging in real time. These results were published in R.A. Janik, J. Jankowski, H. Soltanpanahi, Real-Time dynamics and phase separation in a holographic first order phase transition, Phys. Rev. Lett. 119 (2017) 261601. Subsequent work which gives the details of the numerical simulations, explores various more detailed aspects of this process as well as other classes of theories will appear soon.

String interactions from integrability

As mentioned above, string theory in AdS5xS5 possesses surprising properties of integrability, which enables us to obtain exact results e.g. on the spectrum of the theory. Since this string theory is dual to the N=4 supersymmetric gauge theory, this translates to exact results for the gauge theory valid at any coupling. In particular, one can quantitatively test the AdS/CFT correspondence by comparing the string theory predictions with direct perturbative computations in gauge theories using conventional Feynman graphs.

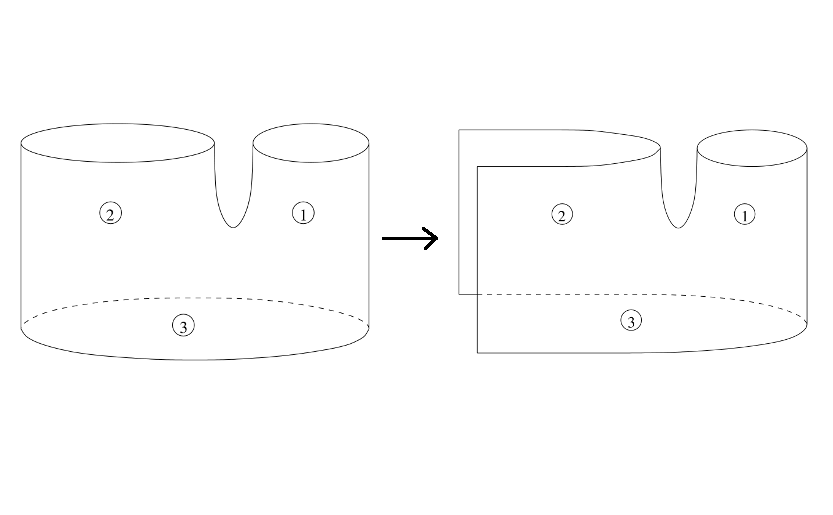
One area where the techniques of integrability were not developed so far was the study of string interactions - e.g. a string splitting into two strings. The relevant splitting amplitude can be also understood as defining the (light-cone) string field theory vertex. In the paper Z. Bajnok, R.A. Janik, String field theory vertex from integrability, JHEP 1504 (2015) 042, we have proposed a completely new way of computing the string vertex through a set of functional equations reminiscent of form factor equations. One arrives at these equations by first decompactifying the ingoing and one of the outgoing strings (see figure) and treating the emission of the third string as a kind of nonlocal form factor.

We have shown how to reproduce the very intricate string vertex for the so-called pp-wave geometry by solving directly these functional equations. In addition, in a subsequent paper we have derived a partial solution (up to a still undetermined form factor) in the general case of strings in AdS5xS5 geometry. The results of further investigations in the classical strong coupling limit are currently in preparation and will appear soon.

Foundational issues in holography

Despite the successes of holography and the many detailed quantitative tests, its precise origin from the field theory perspective remains still elusive. In particular, it would be extremely interesting to understand how gravity emerges in the dual description directly in terms of the original non-gravitational field theory. We are, however, still far from realizing that goal.

In the final part of the grant, we have addressed two questions related to the above issues. Firstly, we took some very basic requirements for a holographic description and constructed their realization for the very simplest possible setting - quantum mechanics of a free particle understood as a 0+1 dimensional quantum field theory. The outcome is a set of three coupled two dimensional topological BF theories. This work was published in R.A. Janik, Towards holography for quantum mechanics, JHEP 1809 (2018) 045.

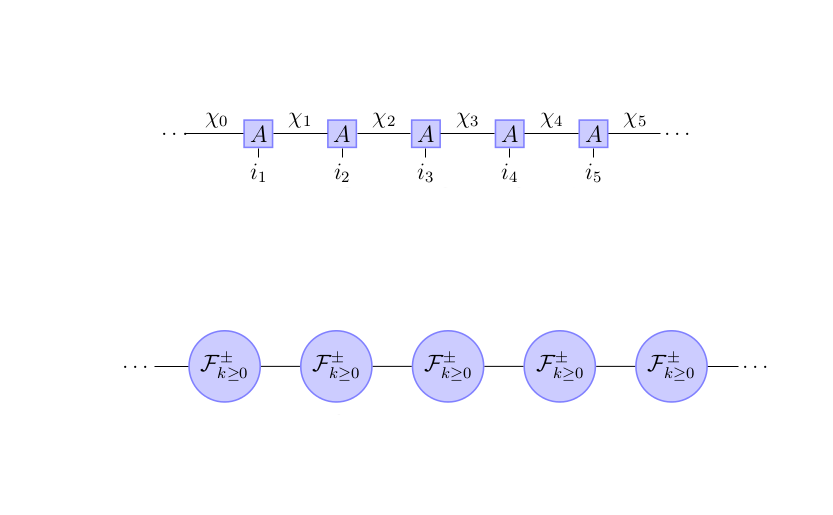
Secondly, we have constructed an exact Matrix Product State (an example of so-called tensor networks) for the ground state of bosonic harmonic chain. The interest in doing this stems from conjectures on the relation of tensor networks and holography. In addition we hypothesized that the infinite dimensional auxillary space (the space of χ's in the figure), necessary in the exact MPS construction could be understood as realizing an emergent dimension. In the very recent work R.A. Janik, Exact bosonic Matrix Product States (and holography), arXiv:1811.11027 we have indeed shown that an infinite dimensional Fock space of states arises at each lattice node. Investigations along both of these lines will be pursued in the future.

Other results

During the course of the project, many other results were obtained. Here we very briefly mention the main areas of research giving links to the arXiv versions of the relevant papers (the ones mentioned previously are given in bold).

Within the part dealing with integrability, issues related to high energy scattering (BFKL pomeron) were investigated in two papers

Three-point correlation functions, string splitting and form factors were investigated from various points of view in Integrability in AdS3/CFT2 correspondence, which exhibits numerous peculiarities and novel phenomena was investigated in Integrability with boundaries was investigated in while some exact string solutions were constructed in

Another line of research dealt with sophisticated methods dealing with asymptotic series appearing in diverse contexts - so-called resurgence. This research tied together the integrability (cusp anomalous dimension) and applied holography research in the grant as well as was developed for its own sake. The related papers are

An extended review on these topics is available at

The applied holography part of the project dealt predominantly with out of equilibrium behaviour, both from the point of view of deviations from hydrodynamics as well as the appearance of novel features in nonconformal theories and in theories with phase transitions. Apart from the Hydrodynamic gradient expansion... paper already mentioned above, this series includes

A second line of research in this part of the project dealt with phenomena associated with defects, constructions of dual holographic solutions, quenches, entanglement observables etc.: The holographic computation of entanglement entropy involves finding certain extremal hypersurfaces in the curved bulk geometry. Their study in a very general context, together with diverse physical applications is presented in a series of papers:

Finally, we moved in the direction of investigating the underlying foundations of holography as already described above. The relevant papers are



NCN Maestro grant 2012/06/A/ST2/00396 From string theory to gauge theory - the AdS/CFT correspondence